2020 Nov P2 Q8.2

Question 8.2

$8.2$	In $\Delta ABC$ , $F$ and $G$ are points on sides $AB$ and $AC$ respectively. $D$ is a point on $GC$ such that $\hat D_1=\hat B$ .

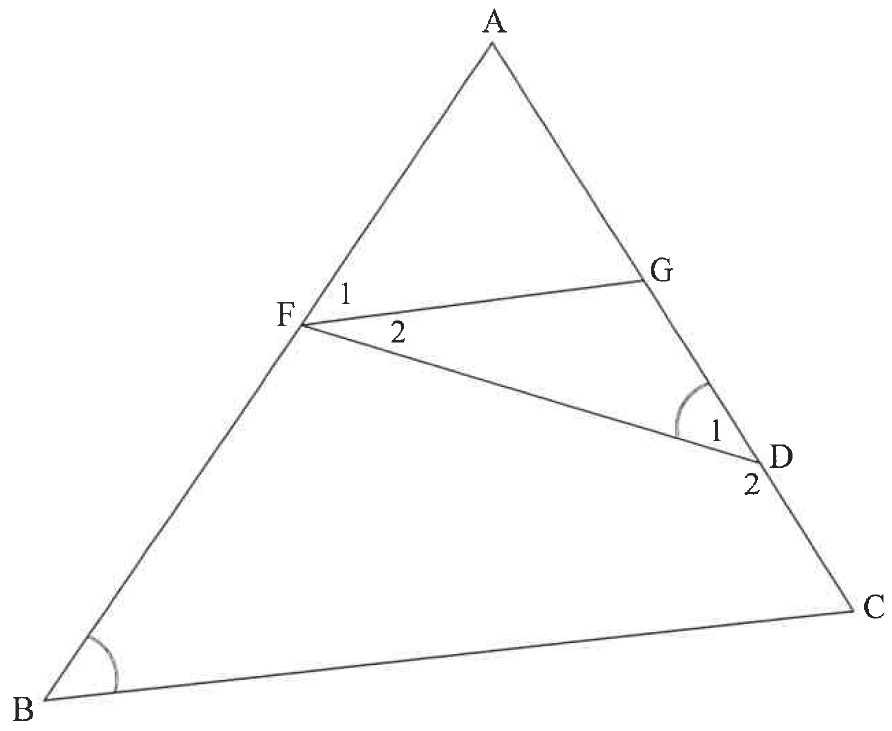$\quad 8.2.1$	If $AF$ is a tangent to the circle passing through points $F$ , $G$ and $D$ , then prove, giving reasons, that $FG\parallel BC$ .	$(4)$
$\quad 8.2.2$	If it is further given that $\frac{\displaystyle AF}{\displaystyle FB}=\frac{\displaystyle 2}{\displaystyle 5}$ , $AC=2x-6$ and $GC=x+9$ , then calculate the value of $x$ .	$(4)$
		$\textbf{[8]}$