2019 Nov P1 Q5

Question 4

$4.1$	Given: $h(x)=\frac{\displaystyle -3}{\displaystyle x-1}+2$
$\quad 4.1.1$	Write down the equations of the asymptotes of $h$ .	$(2)$
$\quad 4.1.2$	Determine the equation of the axis of symmetry of $h$ that has a negative gradient.	$(2)$
$\quad 4.1.3$	Sketch the graph of $h$ , showing the asymptotes and the intercepts with the axes.	$(4)$
$4.2$	The graphs of $\: f(x)=\frac{\displaystyle 1}{\displaystyle 2}(x+5)^2-8$ and $\: g(x)=\frac{\displaystyle 1}{\displaystyle 2}x+\frac{\displaystyle 9}{\displaystyle 2}$ are sketched below. $A$ is the turning point of $f$ . The axis of symmetry of $f$ intersects the $x$ -axis at $E$ and the line $g$ at $D(m\,;\,n)$ . $C$ is the $y$ -intercept of $f$ and $g$ .

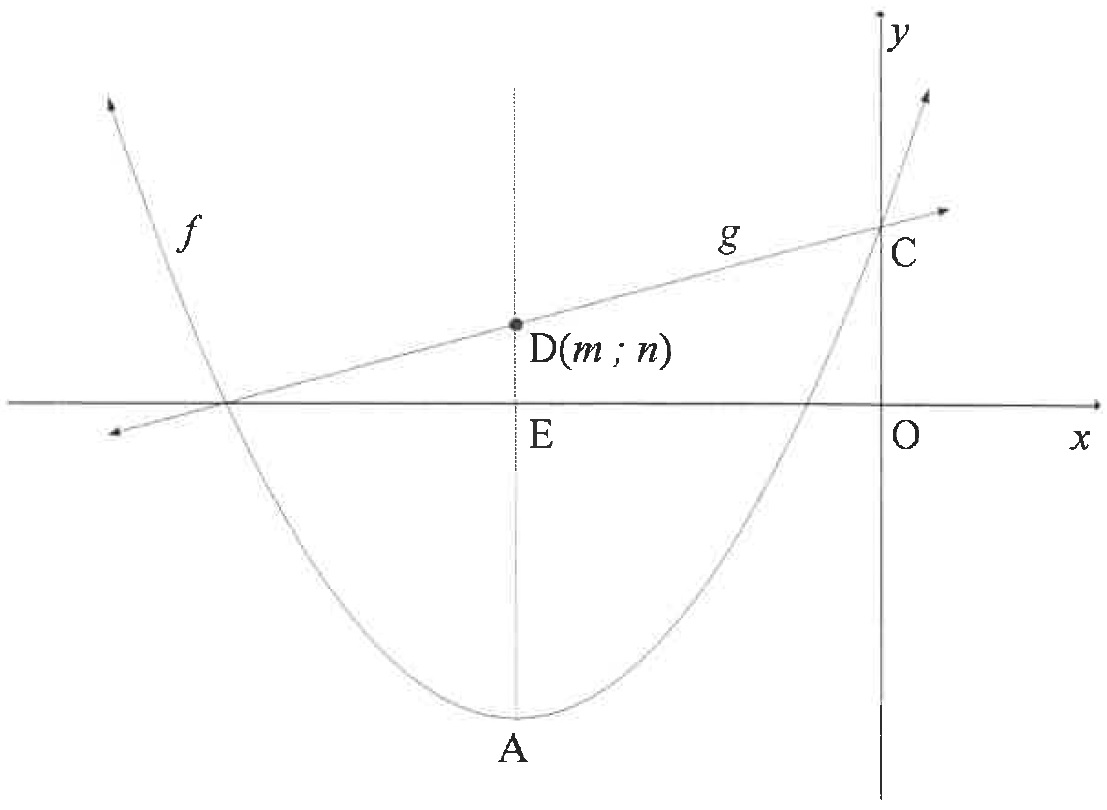$\quad 4.2.1$	Write down the coordinates of $A$ .	$(2)$
$\quad 4.2.2$	Write down the range of $f$ .	$(1)$
$\quad 4.2.3$	Calculate the values of $m$ and $n$ .	$(3)$
$\quad 4.2.4$	Calculate the area of $OCDE$ .	$(3)$
$\quad 4.2.5$	Determine the equation of $g^{-1}$ , the inverse of $g$ , in the form $y=...$	$(2)$
$\quad 4.2.6$	If $h(x)=g^{-1}(x)+k \:$ is a tangent to $f$ , determine the coordinates of the point of contact between $h$ and $f$ .	$(4)$
		$\textbf{[23]}$

The graph of $\,f(x)=3^{-x}\,$ is sketched below. $A$ is the $y$ -intercept of $f$ . $B$ is the point of intersection of $f$ and the line $y=9$ .

$5.1$	Write down the coordinates of $A$ .	$(1)$
$5.2$	Determine the coordinates of $B$ .	$(3)$
$5.3$	Write down the domain of $f^{-1}$ .	$(2)$
$5.4$	Describe the translation from $f$ to $h(x)=\frac{\displaystyle 27}{\displaystyle 3^x}$ .	$(3)$
$5.5$	Determine the values of $x$ for which $h(x)<1$ .	$(3)$
		$\textbf{[12]}$

Sketched below is the graph of $\,f(x)=k^x\,;\,k>0$ . The point $(4\; ;\,16)$ lies on $f$ .

$5.1$	Determine the value of $k$ .	$(2)$
$5.2$	Graph $g$ is obtained by reflecting graph $f$ about the line $y=x$ . Determine the equation of $g$ in the form $y=...$	$(2)$
$5.3$	Sketch the graph $g$ . Indicate on your graph the coordinates of two points on $g$ .	$(4)$
$5.4$	Use your graph to determine the value(s) of $x$ for which:
$\quad 5.4.1$	$f(x) \times g(x)>0$	$(2)$
$\quad 5.4.2$	$g(x) \leq -1$	$(2)$
$5.5$	If $h(x)=f(-x)$ , calculate the value of $x$ for which $f(x)-h(x)=\frac{\displaystyle 15}{\displaystyle 4}$	$(4)$
		$\textbf{[16]}$